For each of the following statements state whether true(T) or false(F).

(ii) True
Two circles of any radii are similar to each other.

(i) False
Two rectangles are similar if their corresponding sides are proportional.

(iii) Falase
If two traingles are similar, the their corresponding angles are equal and their corresponding sides are proportional.


(iv) True
Suppose ABC is a triangle and M, N are

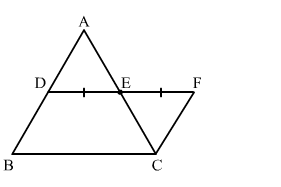
Construction: DE is expanded to F sich that EF = DE

To Prove $=\mathrm{DE}=\frac{1}{2} \mathrm{BC}$

Proof: In △ADE and △CEF
AE = EC        (E is the mid point of AC)
DE = EF        (By construction)
AED = CEF      (Vertically Opposite angle)
By SAS criterion, △ADE ≅ △CEF
CF = AD   (CPCT)
⇒ BD = CF
∠ADE = ∠EFC    (CPCT)
Since, ∠ADE and ∠EFC are alternate angle
Hence, AD ∥ CF and BD ∥ CF
When two sides of a quadrilateral are parallel, then it is a parallelogram
∵DF = BC and BD ∥ CF
∴BDFC is a parallelogram
Hence, DF = BC
⇒ DE + EF = BC

$\Rightarrow \mathrm{DE}=\frac{1}{2} \mathrm{BC}$

(v) False
In △ABC, AB = 6 cm, ∠A = 45∘ and AC = 8 cm and in △DEF, DF = 9 cm, ∠D = 45∘ and DE = 12 cm, then △ABC ∼ △DEF.

In △ABC and △DEF
∠A = ∠D = 45∘ 

$\frac{\mathrm{AB}}{\mathrm{AC}} \neq \frac{\mathrm{DE}}{\mathrm{DF}}$

So △ABC is not similar to △DEF

(vi) False
The polygon formed by joining the mid points of the sides of a quadrilateral is a parallelogram.

(vii) True

Given: △ABC ∼ △DEF

To Prove $=\frac{\operatorname{Ar}(\triangle \mathrm{ABC})}{\mathrm{Ar}(\triangle \mathrm{DEF})}=\left(\frac{\mathrm{AP}}{\mathrm{DQ}}\right)^{2}$

Proof: In △ABP and △DEQ
∠BAP = ∠EDQ        (As ∠A = ∠D, so their Half is also equal)
∠B = ∠E                   (△ABC ∼ △DEF)
By AA criterion, △ABP ∼ △DEQ

$\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{AP}}{\mathrm{DQ}}$   ...........(i)

Since, △ABC ∼ △DEF

$\therefore \frac{\mathrm{Ar}(\triangle \mathrm{ABC})}{\mathrm{Ar}(\triangle \mathrm{DEF})}=\left(\frac{\mathrm{AB}}{\mathrm{DE}}\right)^{2}$

$\Rightarrow \frac{\operatorname{Ar}(\triangle \mathrm{ABC})}{\operatorname{Ar}(\triangle \mathrm{DEF})}=\left(\frac{\mathrm{AP}}{\mathrm{DQ}}\right)^{2} \quad[\mathrm{Using}(1)]$

(viii) True

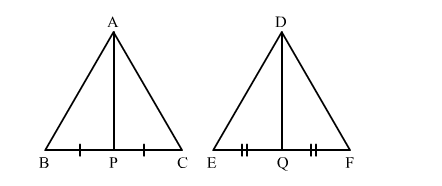
Given: △ABC ∼ △DEF

To Prove $=\frac{\text { Perimeter }(\triangle \mathrm{ABC})}{\text { Perimeter }(\triangle \mathrm{DEF})}=\frac{\mathrm{AP}}{\mathrm{DQ}}$

Proof: In △ABP and △DEQ
∠B = ∠E                  (∵△ABC ∼ △DEF)
∵△ABC ∼ △DEF

$\therefore \frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BC}}{\mathrm{EF}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{DE}}=\frac{2 \mathrm{BP}}{2 \mathrm{EQ}}$

$\Rightarrow \frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BP}}{\mathrm{EQ}}$

By SAS criterion, △ABP ∼ △DEQ

$\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{AP}}{\mathrm{DQ}}$      .....(1)

Since, △ABC ∼ △DEF

$\therefore \frac{\text { Perimeter }(\triangle \mathrm{ABC})}{\text { Perimeter }(\triangle \mathrm{DEF})}=\frac{\mathrm{AB}}{\mathrm{DE}}$

$\Rightarrow \frac{\text { Perimeter }(\triangle \mathrm{ABC})}{\text { Perimeter }(\triangle \mathrm{DEF})}=\frac{\mathrm{AP}}{\mathrm{DQ}} \quad[\mathrm{U} \sin g(1)]$

(ix) True

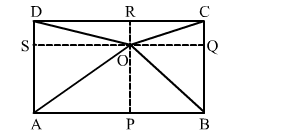
Suppose ABCD is a rectangle with O is any point inside it.
Construction: Join OA, OB, OC, OD and draw two parallel lines SQ ∥ AB ∥ DC and PR ∥ BC ∥ AD
To prove: OA2 + OC2 = OB2 + OD2 
Proof:
OA2 + OC2 = (AS2 + OS2) + (OQ2 + QC2)             [Using Pythagoras theorem in right triangle AOP and COQ]
= (BQ2 + OS2) + (OQ2 + DS2) 
= (BQ2 + OQ2) + (OS2 + DS2)                                 [Using Pythagoras theorem in right triangle BOQ and DOS]
= OB2 + OD2
Hence, LHS = RHS

(x) True

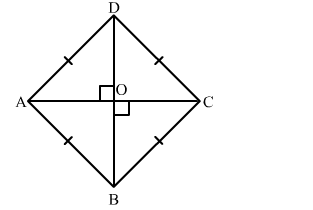
Suppose ABCD is a rhombus having AC and BD its diagonals.
Since, the diagonals of a rhombus perpendicular bisect each other.
Hence, AOC is a right angle triangle
In right triangle AOC
By using Pythagoras theorem, we have

$\mathrm{AB}^{2}=\mathrm{AO}^{2}+\mathrm{OB}^{2}$

$\Rightarrow \mathrm{AB}^{2}=\left(\frac{\mathrm{AC}}{2}\right)^{2}+\left(\frac{\mathrm{BD}}{2}\right)^{2} \quad[\because$ Diagonals of a rhombus perpendicularly bisect each other $]$

$\Rightarrow \mathrm{AB}^{2}=\frac{\mathrm{AC}^{2}}{4}+\frac{\mathrm{BD}^{2}}{4}$

$\Rightarrow 4 \mathrm{AB}^{2}=\mathrm{AC}^{2}+\mathrm{BD}^{2}$

$\Rightarrow \mathrm{AB}^{2}+\mathrm{AB}^{2}+\mathrm{AB}^{2}+\mathrm{AB}^{2}=\mathrm{AC}^{2}+\mathrm{BD}^{2}$

$\Rightarrow \mathrm{AB}^{2}+\mathrm{BC}^{2}+\mathrm{CD}^{2}+\mathrm{DA}^{2}=\mathrm{AC}^{2}+\mathrm{BD}^{2} \quad[\because$ All sides of a rhombus are equal $]$