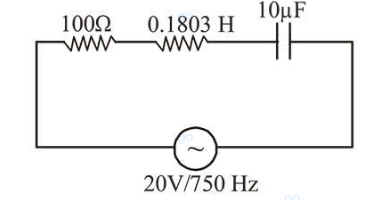
A $750 \mathrm{~Hz}, 20 \mathrm{~V}$ (rms) source is connected to a resistance of $100 \Omega$, an inductance of $0.1803 \mathrm{H}$ and a capacitance of $10 \mu \mathrm{F}$ all in series. The time in which the resistance (heat capacity $2 \mathrm{~J} /{ }^{\circ} \mathrm{C}$ ) will get heated by $10^{\circ} \mathrm{C}$. (assume no loss of heat to the surroundings) is close to :
Correct Option: , 4
(4)
Here, $R=100, X_{L}=L \omega=0.1803 \times 750 \times 2 \pi=850 \Omega$,
$X_{C}=\frac{1}{C \omega}=\frac{1}{10^{-5} \times 2 \pi \times 750}=21.23 \Omega$
Impedance $Z=\sqrt{R^{2}+\left(X_{L}-X_{C}\right)^{2}}$
$=\sqrt{100^{2}+(850-21.23)^{2}}=834.77 \simeq 835$
$H=i_{\mathrm{rms}}^{2} R t=\left(\frac{V_{\mathrm{rms}}}{|Z|}\right)^{2} R T=(m s) \Delta t$
$\Rightarrow \frac{20}{835} \times \frac{20}{835} \times 100 t=(2) \times 10$
$\because V_{\text {rms }}=20 \mathrm{~V}$ and $\Delta \mathrm{t}=10^{\circ} \mathrm{C}$
$\therefore$ Time, $t=348.61 \mathrm{~s}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.