A chord of a circle of radius 10 cm subtends a right angle at the centre.

Here, the radius of the circle is r = 10 cm.

Sector angle of the minor sector made corresponding to the chord AB is 90° 

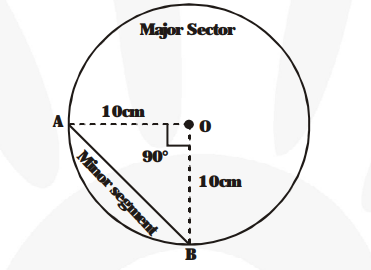
Now, the area of the minor sector $=\frac{\mathbf{9 0}}{\mathbf{3 6 0}} \times \pi \mathrm{r}^{2}$

$=\frac{1}{4} \times \pi \times(10)^{2} \mathrm{~cm}^{2}=\frac{1}{4} \times 3.14 \times 100 \mathrm{~cm}^{2}$

$=\frac{\mathbf{3 1 4}}{\mathbf{4}} \mathbf{c m}^{2}=78.5 \mathrm{~cm}^{2}$

Then, the area of the minor segment

= The area of the minor sector

- The area of the $\Delta \mathrm{OAB}$

$=78.5 \mathrm{~cm}^{2}-\frac{1}{\mathbf{2}} \times \mathrm{OA} \times \mathrm{OB}\left(\because \angle \mathrm{AOB}=90^{\circ}\right)$

$=78.5 \mathrm{~cm}^{2}-\frac{\mathbf{1}}{\mathbf{2}} \times 10 \times 10 \mathrm{~cm}^{2}$

$=(78.5-50) \mathrm{cm}^{2}=28.5 \mathrm{~cm}^{2}$

The area of the major sector

$=\left(\frac{\mathbf{3 6 0}-\mathbf{9 0}}{\mathbf{3 6 0}}\right) \times \pi r^{2}=\frac{\mathbf{2 7 0}}{\mathbf{3 6 0}} \times 3.14 \times(10)^{2} \mathrm{~cm}^{2}$

$=\frac{\mathbf{3}}{\mathbf{4}} \times 314 \mathrm{~cm}^{2}=\frac{\mathbf{3} \times \mathbf{1 5 7}}{\mathbf{2}} \mathbf{c m}^{2}=235.5 \mathrm{~cm}^{2}$