Question:
A chord PQ of a circle is parallel to the tangent drawn at a point R of the circle. Prove that R bisects the arc PRQ.
Solution:
Given Chord PQ is parallel to tangent at R.
To prove R bisects the arc PRQ
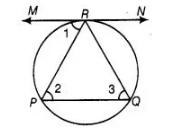
Proof $\angle 1=\angle 2$ [alternate interior angles]
$\angle 1=\angle 3$
[angle between tangent and chord is equal to angle made by chord in alternate segment]
$\therefore$ $\angle 2=\angle 3$
$P R=Q R \quad$ sides opoosite to equal angles are equal
$\Rightarrow \quad P R=Q R$
So, $R$ bisects $P Q$.