Question:
A quadrilateral ABCD is drawn to circumscribe a circle (see fig.).
Prove that AB + CD = AD + BC.
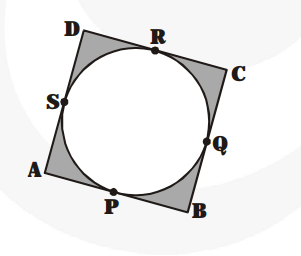
Solution:
In fig., we observe that
$\mathrm{AP}=\mathrm{AS}$ ...(1)
$\{\because \mathrm{AP}$ and $\mathrm{AS}$ are tangents to the circle drawn from the point $\mathrm{A}\}$
Similarly, $\quad B P=B Q \quad \ldots(2)$
$\mathrm{CR}=\mathrm{CQ} \quad \ldots(3)$
$\mathrm{DR}=\mathrm{DS} \quad \ldots(4)$
Adding (1), (2), (3), (4), we have
$(\mathrm{AP}+\mathrm{BP})+(\mathrm{CR}+\mathrm{DR})=(\mathrm{AS}+\mathrm{DS})+(\mathrm{BQ}+\mathrm{CQ})$
$\Rightarrow \mathrm{AB}+\mathrm{CD}=\mathrm{AD}+\mathrm{BC}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
