A rod of mass $M$ and length $L$ is lying on a horizontal frictionless surface. A particle of mass ' $m$ ' travelling along the surface hits at one end of the rod with a velocity ' $u$ ' in a direction perpendicular to the rod. The collision is completely elastic. After collision, particle comes
to rest. The ratio of masses $\left(\frac{\mathrm{m}}{\mathrm{M}}\right)$ is $\frac{1}{\mathrm{x}}$. The value
of ' $x$ ' will be_______.
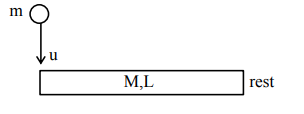
Just before collision

Just after collision
From momentum conservation, $\mathrm{P}_{\mathrm{i}}^{0}=\mathrm{P}_{\mathrm{f}}$
$\mathrm{mu}=\mathrm{Mv}$ $\ldots .$ (i)
From angular momentum conservation about $\mathrm{O}$,
$\mathrm{mu} \cdot \frac{\mathrm{L}}{2}=\frac{\mathrm{ML}^{2}}{12} \omega$
$\Rightarrow \omega=\frac{6 \mathrm{mu}}{\mathrm{ML}}$ $\ldots$ (ii)
From $\mathrm{e}=\frac{\mathrm{R} . \mathrm{V.S}}{\mathrm{R} . \mathrm{V} . \mathrm{A}}$
$1=\frac{\mathrm{V}+\frac{\omega \mathrm{L}}{2}}{\mathrm{u}}$
$\mathrm{v}+\frac{\omega \mathrm{L}}{2}=\mathrm{u}$
$\mathrm{v}+\frac{3 \mathrm{mu}}{\mathrm{M}}=\mathrm{u}$
$\frac{\mathrm{mu}}{\mathrm{M}}+\frac{3 \mathrm{mu}}{\mathrm{M}}=\mathrm{u}$
$\frac{4 \mathrm{mu}}{\mathrm{M}}=\mathrm{u}$
$\frac{m}{M}=\frac{1}{4}$
$X=4$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
