A straight line $\mathrm{L}$ at a distance of 4 units from the origin makes positive intercepts on the coordinate axes and the perpendicular from the origin to this line makes an angle of $60^{\circ}$ with the line $\mathrm{x}+\mathrm{y}=0$. Then an equation of the line $\mathrm{L}$ is :
Correct Option: 1
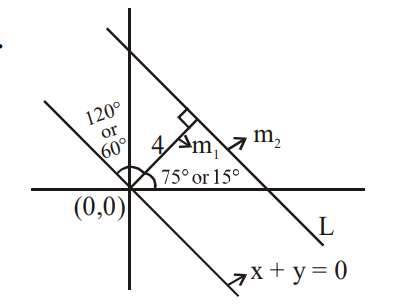
$\mathrm{m}_{1}=\tan 75^{\circ}=\frac{\sqrt{3}+1}{\sqrt{3}-1}$
or $m=\tan 15^{\circ}=\frac{\sqrt{3}-1}{\sqrt{3}+1}$
$\mathrm{m}_{2}=\frac{-1}{\mathrm{~m}_{1}}=\frac{-(\sqrt{3}-1)}{\sqrt{3}+1}$
or $\mathrm{m}_{2}=\frac{-1}{\mathrm{~m}_{1}}=\frac{-(\sqrt{3}+1)}{\sqrt{3}-1}$
$\Rightarrow \mathrm{y}=\mathrm{m}_{2} \mathrm{x}+\mathrm{C} \Rightarrow \mathrm{y}=\frac{-(\sqrt{3}-1) \mathrm{x}}{\sqrt{3}+1}+\mathrm{C} \Rightarrow \mathrm{L}$
or $\mathrm{y}=\frac{-(\sqrt{3}+1) \mathrm{x}}{\sqrt{3}-1}+\mathrm{C} \Rightarrow \mathrm{L}$
Distance from origin $=4$
$\therefore\left|\frac{\mathrm{C}}{\sqrt{1+\frac{(\sqrt{3}-1)^{2}}{(\sqrt{3}+1)^{2}}}}\right|=4$ or $\left|\frac{\mathrm{C}}{\sqrt{1+\frac{(\sqrt{3}+1)^{2}}{(\sqrt{3}-1)^{2}}}}\right|=4$
$\Rightarrow \mathrm{C}=\frac{8 \sqrt{2}}{(\sqrt{3}+1)}$ or $\mathrm{C}=\frac{8 \sqrt{2}}{(\sqrt{3}-1)}$
$\Rightarrow(\sqrt{3}-1) y+(\sqrt{3}+1) x=8 \sqrt{2}$
or $(\sqrt{3}-1) x+(\sqrt{3}+1) y=8 \sqrt{2}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
