A tangent and a normal are drawn at the point $\mathrm{P}(2,-4)$ on the parabola $\mathrm{y}^{2}=8 \mathrm{x}$, which meet the directrix of the parabola at the points $A$ and $B$ respectively. If $Q(a, b)$ is a point such that $A Q B P$ is a square, then $2 a+b$ is equal to :
Correct Option: 1
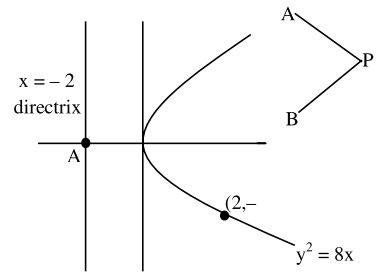
Equation of tangent at $(2,-4)(\mathrm{T}=0)$
$-4 y=4(x+2)$
$x+y+2=0$..(1)
equation of normal
$x-y+\lambda=0$
$\downarrow(2,-4)$
$\lambda=-6$
thus $x-y=6 \ldots(2)$ equation of normal
POI of (1) \& $x=-2$ is $A(-2,0)$
POI of $(2) \& x=-2$ is $\mathrm{A}(-2,8)$
Given $\mathrm{AQBP}$ is a sq.
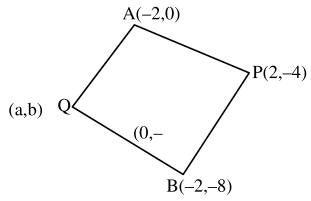
$\Rightarrow \mathrm{m}_{\mathrm{AQ}} \cdot \mathrm{m}_{\mathrm{AP}}=-1$
$\Rightarrow\left(\frac{\mathrm{b}}{\mathrm{a}+2}\right)\left(\frac{4}{-4}\right)=-1 \Rightarrow \mathrm{a}+2=\mathrm{b}$
Also PQ must be parallel to $x$-axis thus
$\Rightarrow \mathrm{b}=-4$
$\therefore \mathrm{a}=-6$
Thus $2 a+b=-16$
27 August 2021, Time 9 AM to 12 PM (Shift 1)
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
