A triangle ABC is drawn to circumscribe a circle of radius 4 cm

Solution:

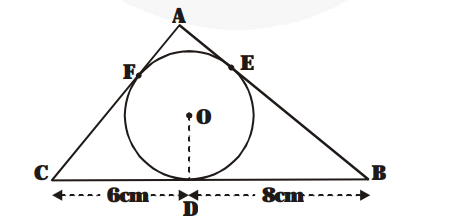
In fig. BD = 8 cm and DC = 6 cm

Then we have $\mathrm{BE}=8 \mathrm{~cm} \quad(\because \mathrm{BE}=\mathrm{BD})$

and $\mathrm{CF}=6 \mathrm{~cm}(\because \mathrm{CF}=\mathrm{CD})$

Suppose $\mathrm{AE}=\mathrm{AF}=\mathrm{x} \mathrm{cm}$

In $\Delta \mathrm{ABC}, \mathrm{a}=\mathrm{BC}=6 \mathrm{~cm}+8 \mathrm{~cm}=14 \mathrm{~cm}$

$\mathrm{b}=\mathrm{CA}=(\mathrm{x}+6) \mathrm{cm}, \mathrm{c}=\mathrm{AB}=(\mathrm{x}+8) \mathrm{cm}$

$\mathrm{s}=\frac{\mathbf{a}+\mathbf{b}+\mathbf{c}}{\mathbf{2}}=\frac{\mathbf{1 4}+(\mathbf{x}+\mathbf{6})+(\mathbf{x}+\mathbf{8})}{\mathbf{2}} \mathbf{c m}$

$=\frac{\mathbf{2 x}+\mathbf{2 8}}{\mathbf{2}} \mathrm{cm}=(\mathrm{x}+14) \mathrm{cm}$

Area of $\triangle \mathrm{ABC}$

$=\sqrt{s(s-a)(s-b)(s-c)}=\sqrt{(x+14) \times x \times 8 \times 6}$

$=\sqrt{48 x \times(x+14)} \mathrm{cm}^{2}$   ...(1)

Also, area of $\Delta \mathrm{ABC}=$ area of $\Delta \mathrm{OBC}+$ area of $\Delta \mathrm{OCA}+$ area of $\Delta \mathrm{OAB}$

$=\frac{1}{2} \times 4 \times a+\frac{1}{2} \times 4 \times b+\frac{1}{2} \times 4 \times c$

$=2(a+b+c)=2 \times 2 s=4 s$

$=4(x+14) \mathrm{cm}^{2}$  ...(2)

From (1) and (2), $\sqrt{48 x \times(x+14)}=4 \times(x+14)$

$\Rightarrow 48 x \times(x+14)=16 \times(x+14)^{2}$

$\Rightarrow 3 \mathrm{x}=\mathrm{x}+14 \quad \Rightarrow \mathrm{x}=7 \mathrm{~cm}$

Then $\mathrm{AB}=\mathrm{c}=(\mathrm{x}+8) \mathrm{cm}=(7+8) \mathrm{cm}=15 \mathrm{~cm}$

and $A C=b=(x+6) c m=(7+6) c m=13 c m$