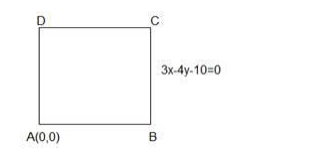
A vertex of a square is at the origin and its one side lies along the line

Given: $A B C D$ is a square and equation of $B C$ is $3 x-4 y-10=0$

To find : Area of the square

Formula used:

We know that the length of perpendicular from $(m, n)$ to the line $a x+b y+c=0$ is given by,

$D=\frac{|a m+b n+c|}{\sqrt{a^{2}+b^{2}}}$

The given equation of the line is $3 x-4 y-10=0$

Here $m=0$ and $n=0, a=3, b=-4, c=-10$

The given equation of the line is $3 x-4 y-10=0$

Here $m=0$ and $n=0, a=3, b=-4, c=-10$

$D=\frac{|3(0)-4(0)-10|}{\sqrt{3^{2}+4^{2}}}$

$D=\frac{|0+0-10|}{\sqrt{9+16}}=\frac{|-10|}{\sqrt{25}}=\frac{|-10|}{5}=\frac{10}{5}=2$

D = 2

Side of the square $=D=2$

Area of the square $=2 \times 2=4$ square units

Area of the square $=4$ square units