AB is a diameter of a circle and C is any point on the circle.

Solution:

Let consider AB be the diameter and C is any point on the circle with radius r.

∠ACB = 90o [angle in the semi-circle is 90o]

Let AC = x

$B C=\sqrt{\bar{A} B^{2}-A C^{2}}$

$\mathrm{BC}=\sqrt{(2 r)^{2}-x^{2}} \Rightarrow \mathrm{BC}=\sqrt{4 r^{2}-x^{2}}$$\ldots(i)$

Now area of $\triangle \mathrm{ABC}, \mathrm{A}=\frac{1}{2} \times \mathrm{AC} \times \mathrm{BC}$

$\Rightarrow A=\frac{1}{2} x \cdot \sqrt{4 r^{2}-x^{2}}$

Squaring on both the sides, we get

$\mathrm{A}^{2}=\frac{1}{4} x^{2}\left(4 r^{2}-x^{2}\right)$

Let $\mathrm{A}^{2}=\mathrm{Z}$

$\mathrm{SO}$ $Z=\frac{1}{4} x^{2}\left(4 r^{2}-x^{2}\right) \Rightarrow Z=\frac{1}{4}\left(4 x^{2} r^{2}-x^{4}\right)$

Differentiating both sides w.r.t. $x$, we get

$\frac{d Z}{d x}=\frac{1}{4}\left[8 x r^{2}-4 x^{3}\right]$ $\ldots(i i)$

For local maxima and local minima $\frac{d Z}{d x}=0$

$x \neq 0 \quad$ and $\quad 2 r^{2}-x^{2}=0$

$\Rightarrow x^{2}=2 r^{2} \Rightarrow x=\sqrt{2} r=\mathrm{AC}$

Now from eq. (i) we have

$\mathrm{BC}=\sqrt{4 r^{2}-2 r^{2}} \Rightarrow \mathrm{BC}=\sqrt{2 r^{2}} \Rightarrow \mathrm{BC}=\sqrt{2} r$

Thus, $\quad A C=B C$

Hence, $\triangle \mathrm{ABC}$ is an isosceles triangle.

Differentiating eq. (ii) w.r.t. $x$, we get $\frac{d^{2} z}{d x^{2}}=\frac{1}{4}\left[8 r^{2}-12 x^{2}\right]$

Put $x=\sqrt{2} r$

$\therefore \frac{d^{2} Z}{d x^{2}}=\frac{1}{4}\left[8 r^{2}-12 \times 2 r^{2}\right]=\frac{1}{4}\left[8 r^{2}-24 r^{2}\right]$

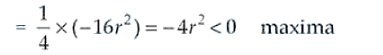
Therefore, the area of Δ ABC is minimum when it is an isosceles triangle.