∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, BDDC=

Given: In $\triangle \mathrm{ABC}, \angle \mathrm{A}=90^{\circ}$ and $\mathrm{AD} \perp \mathrm{BC}$.

To find: BD: DC

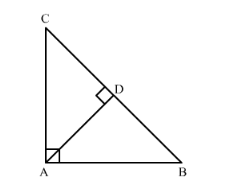
$\angle \mathrm{CAD}+\angle \mathrm{BAD}=90^{\circ} \quad \ldots .1 \angle \mathrm{BAD}+\angle \mathrm{ABD}=90^{\circ} \quad \ldots . .2 \quad \angle \mathrm{ADB}=90^{\circ}$ From $(1)$ and $(2), \angle \mathrm{CAD}=\angle \mathrm{ABD}$

In $\triangle A D B$ and $\triangle A D C$,

$\angle A D B=\angle A D C \quad 90^{\circ}$ each $\angle A B D=\angle C A D \quad$ Proved: $\triangle A D B \sim \triangle A D C \quad$ AA Similarity=CDAD=ACAB=ADBD Corresponding sides are proportional

Disclaimer: The question is not correct. The given ratio cannot be evaluated using the given conditions in the question.