Question.
$A B C D$ is a cyclic quadrilateral whose diagonals intersect at a point $E$. If $\angle D B C=70^{\circ}, \angle B A C$ is $30^{\circ}$, find $\angle B C D$. Further, if $A B=B C$, find $\angle E C D$.
Solution:
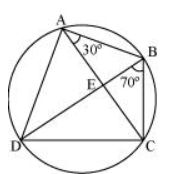
For chord CD,
$\angle C B D=\angle C A D$ (Angles in the same segment)
$\angle C A D=70^{\circ}$
$\angle B A D=\angle B A C+\angle C A D=30^{\circ}+70^{\circ}=100^{\circ}$
$\angle B C D+\angle B A D=180^{\circ}$ (Opposite angles of a cyclic quadrilateral)
$\angle B C D+100^{\circ}=180^{\circ}$
$\angle B C D=80^{\circ}$
$\ln \triangle \mathrm{ABC}$.
$\mathrm{AB}=\mathrm{BC}$ (Given)
$\therefore \angle B C A=\angle C A B$ (Angles opposite to equal sides of a triangle)
$\Rightarrow \angle B C A=30^{\circ}$
We have, $\angle B C D=80^{\circ}$
$\Rightarrow \angle B C A+\angle A C D=80^{\circ}$
$30^{\circ}+\angle \mathrm{ACD}=80^{\circ}$
$\Rightarrow \angle \mathrm{ACD}=50^{\circ}$
$\Rightarrow \angle E C D=50^{\circ}$
For chord CD,
$\angle C B D=\angle C A D$ (Angles in the same segment)
$\angle C A D=70^{\circ}$
$\angle B A D=\angle B A C+\angle C A D=30^{\circ}+70^{\circ}=100^{\circ}$
$\angle B C D+\angle B A D=180^{\circ}$ (Opposite angles of a cyclic quadrilateral)
$\angle B C D+100^{\circ}=180^{\circ}$
$\angle B C D=80^{\circ}$
$\ln \triangle \mathrm{ABC}$.
$\mathrm{AB}=\mathrm{BC}$ (Given)
$\therefore \angle B C A=\angle C A B$ (Angles opposite to equal sides of a triangle)
$\Rightarrow \angle B C A=30^{\circ}$
We have, $\angle B C D=80^{\circ}$
$\Rightarrow \angle B C A+\angle A C D=80^{\circ}$
$30^{\circ}+\angle \mathrm{ACD}=80^{\circ}$
$\Rightarrow \angle \mathrm{ACD}=50^{\circ}$
$\Rightarrow \angle E C D=50^{\circ}$