$A B C D$ is a trapezium in which $A B \| C D, A B=16 \mathrm{~cm}$ and $D C=24 \mathrm{~cm}$. If $E$ and $F$ are respectively the midpoints of $A D$ and $B C$, prove that $\operatorname{ar}(A B F E)=\frac{9}{11} \operatorname{ar}(E F C D)$.
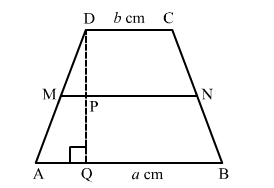
Construction: Draw a perpendicular from point D to the opposite side CD, meeting CD at Q and EF at P.
Let length AQ = h
Given, E and F are the midpoints of AD and BC respectively.
So, $\mathrm{EF}\|\mathrm{AB}\| \mathrm{DC}$ and $\mathrm{EF}=\frac{1}{2}(\mathrm{AB}+\mathrm{DC})=\left(\frac{a+b}{2}\right)$
E is the mid point of AD and EP || AB so by converse of mid point theorem,
EP || DQ and P will be the mid point of AQ.
$\operatorname{ar}(\mathrm{ABFE})=\frac{1}{2} \times \mathrm{AP}(\mathrm{AB}+\mathrm{EF})=\frac{1}{2} h\left(b+\frac{a+b}{2}\right)=\frac{h}{4}(a+3 b)$
$\operatorname{ar}(\mathrm{EFCD})=\frac{1}{2} \times \mathrm{PQ}(\mathrm{CD}+\mathrm{EF})=\frac{1}{2} h\left(a+\frac{a+b}{2}\right)=\frac{h}{4}(b+3 a)$
ar(ABEF) : ar(EFCD) = (a + 3b) : (3a + b)
Here a = 24 cm and b = 16 cm
So, $\frac{\operatorname{ar}(\mathrm{ABEF})}{\operatorname{ar}(\mathrm{EFCD})}=\frac{24+3 \times 16}{16+3 \times 24}=\frac{9}{11}$
