ABCD is a trapezium such that AB and CD are parallel

Question:

$\mathrm{ABCD}$ is a trapezium such that $\mathrm{AB}$ and $\mathrm{CD}$ are parallel and $\mathrm{BC} \perp \mathrm{CD}$. If $\angle \mathrm{ADB}=\theta, \mathrm{BC}=$ $p$ and $C D=q$, then $A B$ is equal to

  1. $\frac{\left(p^{2}+q^{2}\right) \sin \theta}{p \cos \theta+q \sin \theta}$

  2. $\frac{p^{2}+q^{2} \cos \theta}{p \cos \theta+q \sin \theta}$

  3. $\frac{p^{2}+q^{2}}{p^{2} \cos \theta+q^{2} \sin \theta}$

  4. $\frac{\left(p^{2}+q^{2}\right) \sin \theta}{(p \cos \theta+q \sin \theta)^{2}}$

Correct Option: 1,

Solution:

Leave a comment

Close

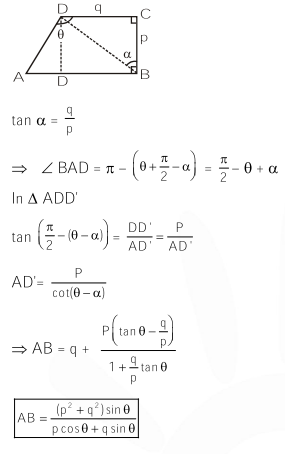
Click here to get exam-ready with eSaral

For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.

Download Now

eSaral Gurukul

NEET

JEE Main

JEE Advanced

Our Telegram Channel

All Study Material