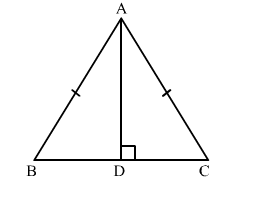
AD is an altitude of an isosceles ΔABC in which AB = AC.

Given: AD is an altitude of an isosceles ΔABC in which AB = AC.

To prove: (i) AD bisects BC, (ii) AD bisects ∠A

Proof:
(i) In ΔABD and ΔACD,

∠">∠∠ADB = ∠">∠∠ADC = 90°">°°          (Given, AD⊥">⊥⊥BC)
AB = AC                                   (Given)
AD = AD                                  (Common side)

∴">∴∴ By RHS congruence criteria,
ΔABD ≅ ΔACD

∴">∴∴ BD = CD       (CPCT)

Hence, AD bisects BC.

(ii)
∵">∵∵ ΔABD ≅ ΔACD       [From (i)]
∴">∴∴ ∠">∠∠BAD = ∠">∠∠CAD      (CPCT)
Hence, AD bisects ∠A.