Area of a sector of central angle 200°

Let the radius of the sector AOBA be r.

Given that, Central angle of sector $A O B A=\theta=200^{\circ}$

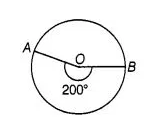
and area of the sector $A O B A=770 \mathrm{~cm}^{2}$

We know that, area of the sector $=\frac{\pi r^{2}}{360^{\circ}} \times \theta^{\circ}$

$\therefore \quad$ Area of the sector, $770=\frac{\pi r^{2}}{360^{\circ}} \times 200$

$\Rightarrow \quad \frac{77 \times 18}{\pi}=r^{2}$

$\Rightarrow \quad r^{2}=\frac{77 \times 18}{22} \times 7 \Rightarrow r^{2}=9 \times 49$

$\Rightarrow \quad r=3 \times 7$

$\therefore \quad r=21 \mathrm{~cm}$

So, radius of the sector $A O B A=21 \mathrm{~cm}$.

Now, the length of the correspoding arc of this sector $=$ Central angle $\times$ Radius

$=200 \times 21 \times \frac{\pi}{180^{\circ}} \quad\left[\because 1^{\circ}=\frac{\pi}{180} R\right]$

$=\frac{20}{18} \times 21 \times \frac{22}{7}$

$=\frac{220}{3} \mathrm{~cm}=73 \frac{1}{3} \mathrm{~cm}$

Hence, the required length of the corresponding arc is $73 \frac{1}{3} \mathrm{~cm}$.