Assume that a tunnel is dug along a chord of the earth, at a perpendicular distance $(\mathrm{R} / 2)$ from the earth's centre, where ' $R$ ' is the radius of the Earth. The wall of the tunnel is frictionless. If a particle is released in this tunnel, it will execute a simple harmonic motion with a time period:
Correct Option: , 4
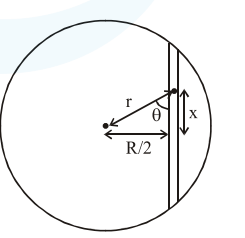
Force along the tunnel
$\mathrm{F}=-\left(\frac{\mathrm{GMmr}}{\mathrm{R}^{3}}\right) \cos \theta$
$\mathrm{F}=-\frac{\mathrm{gm}}{\mathrm{R}} \mathrm{x}\left(\frac{\mathrm{GM}}{\mathrm{R}^{2}}=\mathrm{g}, \mathrm{r} \cos \theta=\mathrm{x}\right)$
$a=-\frac{g}{R} x$
$\omega^{2}=\frac{g}{R} \quad T=2 \pi \sqrt{\frac{R}{g}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
