Question:
Compute the area of trapezium PQRS in figure
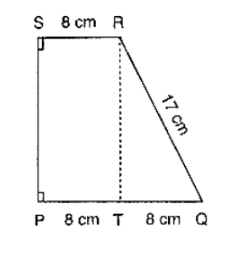
Solution:
We have,
ar(trap. PQRS) = ar(rect. PSRT) + ar(ΔQRT)
⇒ ar(trap. PQRS) = PT × RT + 1/2(QT × RT)
= 8 × RT + 1/2(8 × RT) = 12 × RT
In ΔQRT, we have
$\mathrm{QR}^{2}=\mathrm{QT}^{2}+\mathrm{RT}^{2}$
$\Rightarrow \mathrm{RT}^{2}=\mathrm{QR}^{2}-\mathrm{QT}^{2}$
$\Rightarrow R T^{2}=17^{2}-8^{2}=225$
⇒ RT = 15
Hence, Area of trapezium $=12 \times 15 \mathrm{~cm}^{2}=180 \mathrm{~cm}^{2}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
