Question:
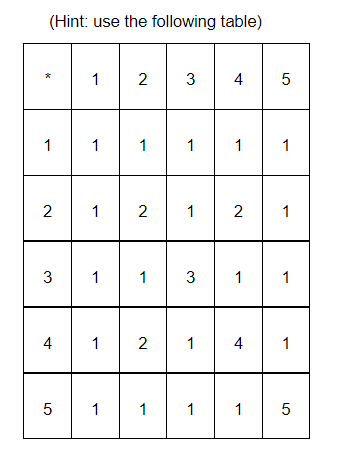
Consider a binary operation * on the set $\{1,2,3,4,5\}$ given by the following multiplication table.
(i) Compute $(2 * 3)^{*} 4$ and $2 *\left(3^{*} 4\right)$
(ii) Is * commutative?
(iii) Compute $\left(2^{*} 3\right)^{*}\left(4^{*} 5\right)$.
Solution:
(i) $\left(2^{*} 3\right)^{*} 4=1^{*} 4=1$
$2^{*}\left(3^{*} 4\right)=2^{*} 1=1$
(ii) For every $a, b \in\{1,2,3,4,5\}$, we have $a^{*} b=b^{*} a$. Therefore, the operation * is commutative.
(iii) $\left(2^{*} 3\right)=1$ and $\left(4^{*} 5\right)=1$
$\therefore(2 * 3)^{*}\left(4^{*} 5\right)=1^{*} 1=1$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
