Consider a water tank as shown in the figure.
It's cross-sectional
area is $0.4 \mathrm{~m}^{2}$. The tank has an opening B near the bottom whose cross-section area is $1 \mathrm{~cm}^{2}$. A load of $24 \mathrm{~kg}$ is applied on the water at the top when the height of the water level is $40 \mathrm{~cm}$ above the bottom, the velocity of water coming out the opening $B$ is $v m s^{-1}$. The value of $\mathrm{v}$, to the nearest integer, is [Take value of $\mathrm{g}$ to be $10 \mathrm{~ms}^{-2}$ ]
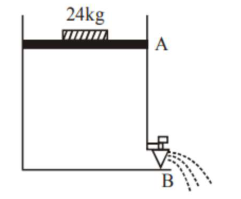
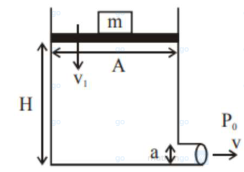
$\mathrm{m}=24 \mathrm{~kg}$
$\mathrm{~A}=0.4 \mathrm{~m}^{2}$
$\mathrm{a}=1 \mathrm{~cm}^{2}$
$\mathrm{H}=40 \mathrm{~cm}$
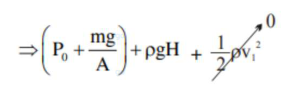
$=\mathrm{P}_{0}+0+\frac{1}{2} \rho \mathrm{v}^{2} \ldots(1)$
$\Rightarrow \mathrm{Neglecting} \mathrm{v}_{1}$
$\Rightarrow \mathrm{v}=\sqrt{2 \mathrm{gH}+\frac{2 \mathrm{mg}}{\mathrm{A} \rho}}$
$\Rightarrow \mathrm{v}=\sqrt{8+1.2}$
$\Rightarrow \mathrm{v}=3.033 \mathrm{~m} / \mathrm{s}$
$\Rightarrow \mathrm{v} \simeq 3 \mathrm{~m} / \mathrm{s}$
