Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC). Prove that ABCD is a trapezium.

Solution:



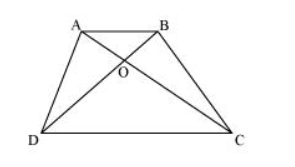
It is given that

Area $(\triangle \mathrm{AOD})=$ Area $(\Delta \mathrm{BOC})$

Area $(\triangle \mathrm{AOD})+$ Area $(\triangle \mathrm{AOB})=$ Area $(\triangle \mathrm{BOC})+$ Area $(\triangle \mathrm{AOB})$

Area $(\triangle A D B)=$ Area $(\triangle A C B)$

We know that triangles on the same base having areas equal to each other lie between the same parallels.

Therefore, these triangles, $\triangle \mathrm{ADB}$ and $\triangle \mathrm{ACB}$, are lying between the same parallels.

i.e., $A B \| C D$

Therefore, $A B C D$ is a trapezium.