Draw a quadrilateral in the Cartesian plane,

Let ABCD be the given quadrilateral with vertices A (–4, 5), B (0, 7), C (5, –5), and D (–4, –2).

Then, by plotting A, B, C, and D on the Cartesian plane and joining AB, BC, CD, and DA, the given quadrilateral can be drawn as

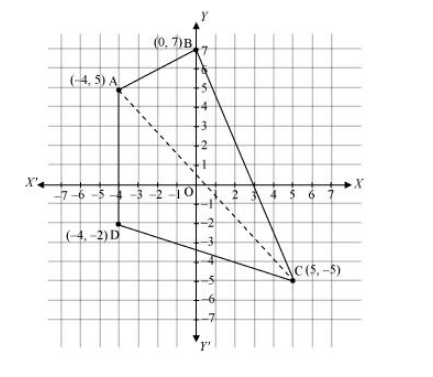
To find the area of quadrilateral ABCD, we draw one diagonal, say AC.

Accordingly, area (ABCD) = area (ΔABC) + area (ΔACD)

$=\frac{1}{2}|-4(7+5)+0(-5-5)+5(5-7)|$ unit $^{2}$

$=\frac{1}{2}|-4(12)+5(-2)|$ unit $^{2}$

$=\frac{1}{2}|-48-10|$ unit $^{2}$

$=\frac{1}{2}|-58|$ unit $^{2}$

$=\frac{1}{2} \times 58$ unit $^{2}$

$=29$ unit $^{2}$

Area of $\triangle \mathrm{ACD}$

$=\frac{1}{2}|-4(-5+2)+5(-2-5)+(-4)(5+5)|$ unit $^{2}$

$=\frac{1}{2}|-4(-3)+5(-7)-4(10)|$ unit $^{2}$

$=\frac{1}{2}|12-35-40|$ unit $^{2}$

$=\frac{1}{2}|-63|$ unit $^{2}$

$=\frac{63}{2}$ unit $^{2}$

Thus, area $(\mathrm{ABCD})=\left(29+\frac{63}{2}\right)$ unit $^{2}=\frac{58+63}{2}$ unit $^{2}=\frac{121}{2}$ unit $^{2}$