Establish the following vector inequalities geometrically or otherwise:

solution:

(a) Let two vectors $\vec{a}$ and $\vec{b}$ be represented by the adjacent sides of a parallelogram OMNP, as shown in the given figure.



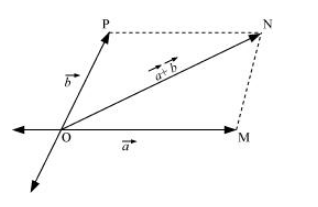
Here, we can write:

$|\overrightarrow{\mathrm{OM}}|=|\vec{a}|$ $\ldots(i)$

$|\overrightarrow{\mathrm{MN}}|=|\overrightarrow{\mathrm{OP}}|=|\vec{b}|$ $\ldots(i i)$

$|\overrightarrow{\mathrm{ON}}|=|\vec{a}+\vec{b}|$ $\ldots(i i i)$

in a triangle, each side is smaller than the sum of the other two sides.

Therefore, in $\Delta O M N$, we have:

$O N<(O M+M N)$

$|\vec{a}+\vec{b}|<|\vec{a}|+|\vec{b}|$ $\ldots(i v)$

If the two vectors $\vec{a}$ and $\vec{b}$ act along a straight line in the same direction, then we can write:

$|\vec{a}+\vec{b}|=|\vec{a}|+|\vec{b}|$ $\ldots(v)$

Combining equations (iv) and $(v)$, we get:

$|\vec{a}+\vec{b}| \leq|\vec{a}|+|\vec{b}|$

(b) Let two vectors $\vec{a}$ and $\vec{b}$ be represented by the adjacent sides of a parallelogram OMNP, as shown in the given figure.



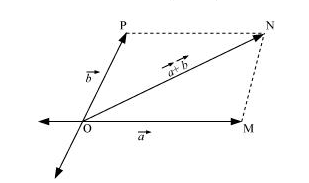
Here, we have:

$|\overrightarrow{\mathrm{OM}}|=|\vec{a}|$ $\ldots(i)$

$|\overrightarrow{\mathrm{MN}}|=|\overrightarrow{\mathrm{OP}}|=|\vec{b}|$ $\ldots(i i)$

$|\overrightarrow{\mathrm{ON}}|=|\vec{a}+\vec{b}|$ $\ldots(i i i)$

In a triangle, each side is smaller than the sum of the other two sides.

Therefore, in $\triangle \mathrm{OMN}$, we have:

$\mathrm{ON}+\mathrm{MN}>\mathrm{OM}$

$\mathrm{ON}+\mathrm{OM}>\mathrm{MN}$

$|\overrightarrow{\mathrm{ON}}|>|\overrightarrow{\mathrm{OM}}-\overrightarrow{\mathrm{OP}}|$ $(\because \mathrm{OP}=\mathrm{MN})$

$|\vec{a}+\vec{b}|>\| \vec{a}|-| \vec{b}|| \ldots$ (iv)

If the two vectors $\vec{a}$ and $\vec{b}$ act along a straight line in the opposite direction, then we can write:

$|\vec{a}+\vec{b}|=|| \vec{a}|-| \vec{b}|| \ldots$ $(v)$

Combining equations (iv) and (v), we get:

$|\vec{a}+\vec{b}| \geq|| \vec{a}|-| \vec{b}||$

(c) Let two vectors $\vec{a}$ and $\vec{b}$ be represented by the adjacent sides of a parallelogram PORS, as shown in the given figure.



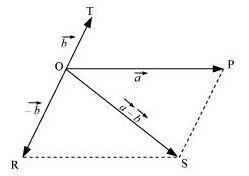
Here we have:

$|\overrightarrow{\mathrm{OR}}|=|\overrightarrow{\mathrm{PS}}|=|\vec{b}|$ $\ldots(i)$

$|\overrightarrow{\mathrm{OP}}|=|\vec{a}|$ $\ldots(i i)$

In a triangle, each side is smaller than the sum of the other two sides. Therefore, in $\Delta \mathrm{OPS}$, we have:

$\mathrm{OS}<\mathrm{OP}+\mathrm{PS}$

$|\vec{a}-\vec{b}|<|\vec{a}|+|-\vec{b}|$

$|\vec{a}-\vec{b}|<|\vec{a}|+|\vec{b}|$ $\ldots(i i i)$

If the two vectors act in a straight line but in opposite directions, then we can write:

$|\vec{a}-\vec{b}|=|\vec{a}|+|\vec{b}| \ldots$ (iv)

Combining equations (iii) and (iv), we get:

$|\vec{a}-\vec{b}| \leq|\vec{a}|+|\vec{b}|$

(d) Let two vectors $\vec{a}$ and $\vec{b}$ be represented by the adjacent sides of a parallelogram PORS, as shown in the given figure.



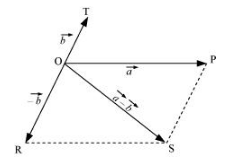
The following relations can be written for the given parallelogram.

$\mathrm{OS}+\mathrm{PS}>\mathrm{OP}$ $\ldots(i)$

$\mathrm{OS}>\mathrm{OP}-\mathrm{PS}$ ... (ii)

$|\vec{a}-\vec{b}|>|\vec{a}|-|\vec{b}|$ $\ldots$ (iii)

The quantity on the LHS is always positive and that on the RHS can be positive or negative. To make both quantities positive, we take modulus on both sides as:

||$\vec{a}-\vec{b}||>|| \vec{a}|-| \vec{b}||$

$|\vec{a}-\vec{b}|>|| \vec{a}|-| \vec{b}||$ $\ldots(i v)$

If the two vectors act in a straight line but in the same directions, then we can write:

$|\vec{a}-\vec{b}|=|| \vec{a}|-| \vec{b}||$ $\ldots(v)$

Combining equations (iv) and (v), we get:

$|\vec{a}-\vec{b}| \geq|| \vec{a}|-| \vec{b}||$