Find the area of a parallelogram ABCD if three of its vertices

It is given that $\mathrm{A}(2,4), \mathrm{B}(2+\sqrt{3}, 5)$ and $\mathrm{C}(2,6)$ are the vertices of the parallelogram $\mathrm{ABCD}$.

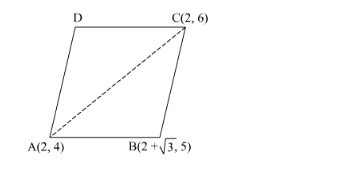
We know that the diagonal of a parallelogram divides it into two triangles having equal area.

$\therefore$ Area of the parallogram $\mathrm{ABCD}=2 \times$ Area of the $\triangle \mathrm{ABC}$

Now,

$\operatorname{ar}(\Delta \mathrm{ABC})=\frac{1}{2}\left|x_{1}\left(y_{2}-y_{3}\right)+x_{2}\left(y_{3}-y_{1}\right)+x_{3}\left(y_{1}-y_{2}\right)\right|$

$=\frac{1}{2}|2(5-6)+(2+\sqrt{3})(6-4)+2(4-5)|$

$=\frac{1}{2}|-2+4+2 \sqrt{3}-2|$

$=\frac{1}{2} \times 2 \sqrt{3}$

$=\sqrt{3}$ square units

$\therefore$ Area of the parallooram $A B C D=2 \times$ Area of the $\triangle A B C=2 \times \sqrt{3}=2 \sqrt{3}$ square units

Hence, the area of given parallelogram is $2 \sqrt{3}$ square units.