Find the area of the shaded region in fig.,

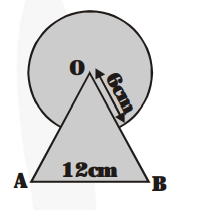
Area of the circle with radius 6 cm

$=\pi \mathrm{r}^{2}=\frac{22}{7} \times 6 \times 6 \mathrm{~cm}^{2}=\frac{792}{7} \mathrm{~cm}^{2}$

Area of equilateral triangle, having side

a = 12 cm, is given by

$\frac{\sqrt{3}}{4} a^{2}=\frac{\sqrt{3}}{4} \times 12 \times 12 \mathrm{~cm}^{2}=36 \sqrt{3} \mathrm{~cm}^{2}$

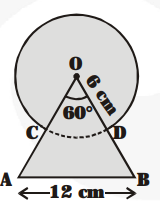
$\because \quad$ Each angle of an equilateral triangle $=60^{\circ}$

$\therefore \quad \angle \mathrm{AOB}=60^{\circ}$

$\therefore$ Area of sector COD

$=\frac{\theta}{\mathbf{3 6 0}^{\circ}} \times \pi^{2}=\frac{\mathbf{6 0}^{\circ}}{\mathbf{3 6 0}^{\circ}} \times \frac{22}{\mathbf{7}} \times 6 \times 6 \mathrm{~cm}^{2}$

$=\frac{22 \times 6}{7} \mathrm{~cm}^{2}=\frac{132}{7} \mathrm{~cm}^{2}$

Now, area of the shaded region,

= [Area of the circle] + [Area of the equilateral triangle] – [Area of the sector COD]

$=\left[\frac{792}{7}+36 \sqrt{3}-\frac{132}{7}\right] \mathrm{cm}^{2}$

$=\left[\frac{\mathbf{6} \mathbf{C D}}{\mathbf{7}}+\mathbf{3 6} \sqrt{\mathbf{3}}\right] \mathrm{cm}^{2}$