Find the area of the triangle formed by joining

Solution:

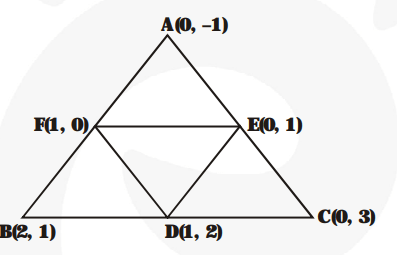
Let the vertices of the triangle be A(0, –1), B(2, 1) and C(0, 3).

Let D, E and F be the mid-points of the sides BC, CA and AB respectively. Then :

Coordinates of D are

$\left(\frac{\mathbf{2}+\mathbf{0}}{\mathbf{2}}, \frac{\mathbf{1}+\mathbf{3}}{\mathbf{2}}\right)$ i.e., $\left(\frac{\mathbf{2}}{\mathbf{2}}, \frac{\mathbf{4}}{\mathbf{2}}\right)$ or $(1,2)$

Coordinates of $\mathrm{E}$ are $\left(\frac{\mathbf{0}+\mathbf{0}}{\mathbf{2}}, \frac{\mathbf{3}+(\mathbf{1})}{\mathbf{2}}\right)$ i.e., $(0,1)$

Coordinates of F are $\left(\frac{\mathbf{2}+\mathbf{0}}{\mathbf{2}}, \frac{\mathbf{1}+(\mathbf{1})}{\mathbf{2}}\right)$ i.e., $(1,0)$



Now, $\operatorname{ar}(\Delta \mathrm{ABC})$

$=\frac{\mathbf{1}}{\mathbf{2}}[0(1-3)+2\{3-(-1)\}+0(-1-1)]$

$=\frac{1}{2}[0(-2)+8+0(-2)]$

$=\frac{\mathbf{1}}{\mathbf{2}}[0+8+0]=\frac{\mathbf{1}}{\mathbf{2}} \times 8=4$ sq. units

Now, ar $(\Delta \mathrm{DEF})=\frac{\mathbf{1}}{\mathbf{2}}[1(1-0)+0(0-2)+1(2-1)]$

$=\frac{1}{2}[1(1)+0+1(1)]$

$=\frac{\mathbf{1}}{\mathbf{2}}[1+0+1]=\frac{\mathbf{1}}{\mathbf{2}} \times 2=1$ sq. unit

$\therefore \frac{\operatorname{ar}(\Delta \mathrm{D}) \mathrm{AF})}{\operatorname{ar}(\triangle \mathrm{ABC})}=\frac{1}{4}$

$\therefore \quad \operatorname{ar}(\Delta \mathrm{DEF}): \operatorname{ar}(\triangle \mathrm{ABC})=1: 4$