Find the condition that the zeros of the polynomial f(x)

Let $a-d, a$ and $a+d$ be the zeros of the polynomials $f(x)$. Then,

Sum of the zeros $=\frac{\text { Coefficient of } x^{2}}{\text { Coefficient of } x^{3}}$

$a-d+a+a+d=\frac{-3 p}{1}$

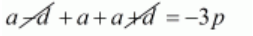
$3 a=-3 p$

$a=\frac{-3 \times p}{3}$

$a=-p$

Since $a$ is a zero of the polynomial $f(x)$. Therefore,

$f(x)=x^{3}+3 p x^{2}+3 q x+r$

$f(a)=0$

$f(a)=a^{3}+3 p a^{2}+3 q a+r$

$a^{3}+3 p a^{2}+3 q a+r=0$

Substituting $a=-p$ we get,

$(-p)^{3}+3 p(-p)^{2}+3 q(-p)+r=0$

$-p^{3}+3 p^{3}-3 p q+r=0$

$2 p^{3}-3 p q+r=0$

Hence, the condition for the given polynomial is $2 p^{3}-3 p q+r=0$.