Find the coordinates of the points of trisection of the line segment joining

Solution:



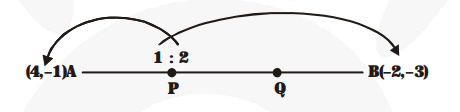
Points P and Q trisect the line segment joining the points A(4, – 1) and B(2, – 3),

i.e., AP = PQ = QB.

Here, P divides AB in the ratio 1 : 2 and Q divides AB in the ratio 2 : 1.

$x$-coordinate of $P=\frac{1 \times(-2)+2 \times(4)}{1+2}=\frac{6}{3}=2$;

$y-$ coordinate of $P=\frac{1 \times(-3)+2 \times(-1)}{1+2}=\frac{-5}{3}$

Thus, the coordinates of $P$ are $(\mathbf{2}, \mathbf{- 5})$.

Now, $x$ coordinate of $Q=\frac{\boldsymbol{2} \times(-\boldsymbol{2})+\mathbf{1 ( 4 )}}{\boldsymbol{2}+\mathbf{1}}=\mathbf{0}$

$y-$ coordinate of $Q=\frac{\mathbf{2} \times(\mathbf{- 3})+\mathbf{1} \times(\mathbf{- 1})}{\mathbf{2}+\mathbf{1}}=-\frac{\mathbf{7}}{\mathbf{3}}$

Thus, the coordinates of $Q$ are $\left(\mathbf{0},-\frac{\mathbf{7}}{\mathbf{3}}\right)$.

Hence, the points of trisection are $P\left(2, \frac{-5}{3}\right)$ and $Q\left(\mathbf{0},-\frac{7}{3}\right)$.