Find the distance between the points (0,0) and (36,15).

Solution:

Part-I

Let the points be A(0, 0) and B(36, 15)

$\therefore \quad \mathrm{AB}=\sqrt{(36-0)^{2}+(15-0)^{2}}$

$=\sqrt{(36)^{2}+(15)^{2}}=\sqrt{1296+225}$

$=\sqrt{\mathbf{1 5 2 1}}=\sqrt{\mathbf{3 9}^{\mathbf{2}}}=39$

Part-II

We have A(0, 0) and B(36, 15) as the positions of two towns



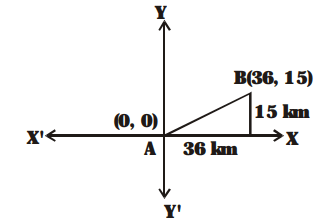
Here $x_{1}=0, x_{2}=36$ and $y_{1}=0, y_{2}=15$

$\therefore \quad \mathrm{AB}=\sqrt{(\mathbf{3 6}-\mathbf{0})^{2}+(\mathbf{1 5}-\mathbf{0})^{2}}=39 \mathrm{~km}$