Find the real values of θ for which

Since $\frac{1+i \cos \theta}{1-2 i \cos \theta}$ is purely real

Firstly, we need to solve the given equation and then take the imaginary part as 0

$\frac{1+i \cos \theta}{1-2 i \cos \theta}$

We rationalize the above by multiply and divide by the conjugate of (1 -2i cos θ)

$=\frac{1+i \cos \theta}{1-2 i \cos \theta} \times \frac{1+2 i \cos \theta}{1+2 i \cos \theta}$

$=\frac{(1+i \cos \theta)(1+2 i \cos \theta)}{(1-2 i \cos \theta)(1+2 i \cos \theta)}$

We know that,

$(a-b)(a+b)=\left(a^{2}-b^{2}\right)$

$=\frac{1(1)+1(2 i \cos \theta)+i \cos \theta(1)+i \cos \theta(2 i \cos \theta)}{(1)^{2}-(2 i \cos \theta)^{2}}$

$=\frac{1+2 i \cos \theta+i \cos \theta+2 i^{2} \cos ^{2} \theta}{1-4 i^{2} \cos ^{2} \theta}$

$=\frac{1+3 i \cos \theta+2(-1) \cos ^{2} \theta}{1-4(-1) \cos ^{2} \theta}\left[\because i^{2}=-1\right]$

$=\frac{1+3 i \cos \theta-2 \cos ^{2} \theta}{1+4 \cos ^{2} \theta}$

$=\frac{1-2 \cos ^{2} \theta}{1+4 \cos ^{2} \theta}+i \frac{3 \cos \theta}{1+4 \cos ^{2} \theta}$

Since $\frac{1+i \cos \theta}{1-2 i \cos \theta}$ is purely real [given]

Hence, imaginary part is equal to 0

i.e. $\frac{3 \cos \theta}{1+4 \cos ^{2} \theta}=0$

$\Rightarrow 3 \cos \theta=0 \times\left(1+4 \cos ^{2} \theta\right)$

$\Rightarrow 3 \cos \theta=0$

$\Rightarrow \cos \theta=0$

$\Rightarrow \cos \theta=\cos 0$

Since, $\cos \theta=\cos y$

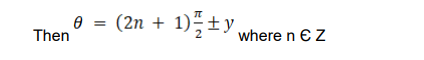
Putting y = 0

$\theta=(2 n+1) \frac{\pi}{2} \pm 0$

$\theta=(2 n+1) \frac{\pi}{2}$ where $n \in Z$

Hence, for $\theta=(2 \mathrm{n}+1) \frac{\pi}{2} .$ where $\mathrm{n} \in \mathrm{Z} \frac{1+\mathrm{i} \cos \theta}{1-2 \mathrm{i} \cos \theta}$ is purely real.