Question:
Find the value of $x$ for which $D E \| A B$ in given figure.
Solution:
Given, $D E \| A B$
$\therefore$ $\frac{C D}{A D}=\frac{C E}{B E}$ [by basic proportionality theorem]
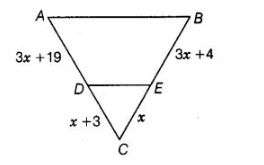
$\Rightarrow \quad \frac{x+3}{3 x+19}=\frac{x}{3 x+4}$
$\Rightarrow \quad(x+3)(3 x+4)=x(3 x+19)$
$\Rightarrow \quad 19 x-13 x=12$
$\Rightarrow \quad 6 x=12$
$\therefore$ $x=\frac{12}{6}=2$
Hence, the required value of $x$ is 2 .
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
