From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in fig.

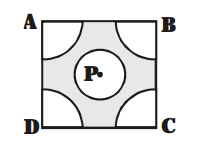
Side of the square = 4 cm

$\therefore$ Area of the square $\mathrm{ABCD}=4 \times 4 \mathrm{~cm}^{2}$

$=16 \mathrm{~cm}^{2}$

$\because$ Each corner has a quadrant circle of radius $1 \mathrm{~cm}$.

$\therefore \quad$ Area of all the 4 quadrant squares

$4 \times \frac{1}{4} \pi r^{2}=\pi r^{2}=\frac{22}{7} \times 1 \times 1 \mathrm{~cm}^{2}=\frac{22}{7} \mathrm{~cm}^{2}$

Diameter of the middle circle = 2 cm

$\Rightarrow$ Radius of the middle circle $=1 \mathrm{~cm}$

$\therefore \quad$ Area of the middle circle

$=\pi \mathrm{r}^{2}=\frac{22}{7} \times 1 \times 1 \mathrm{~cm}^{2}=\frac{22}{7} \mathrm{~cm}^{2}$

Now, area of the shaded region

= [Area of the square ABCD] – [(Area of the 4 quadrant circles) + (Area of the middle circle)]

$=\left[16 \mathrm{~cm}^{2}\right]-\left[\left(\frac{\text { 22 }}{\boldsymbol{7}}+\frac{\text { 22 }}{\boldsymbol{7}}\right) \mathbf{c m}^{2}\right]$

$=16 \mathrm{~cm}^{2}-2 \times \frac{22}{7} \mathrm{~cm}^{2}$

$=16 \mathrm{~cm}^{2}-\frac{44}{7} \mathrm{~cm}^{2}=\frac{112-44}{7} \mathrm{~cm}^{2}=\frac{68}{7} \mathrm{~cm}^{2}$