Question:
If in two Δ DEF and Δ PQR,∠D =∠Q and ∠R = ∠E,then which of the following is not true?
(a) $\frac{E F}{P R}=\frac{D F}{P Q}$
(b) $\frac{D E}{P Q}=\frac{E F}{R P}$
(c) $\frac{D E}{Q R}=\frac{D F}{P Q}$
(d) $\frac{E F}{R P}=\frac{D E}{Q R}$
Solution:
(b) Given,in ΔDEF,∠D =∠Q,∠R = ∠E
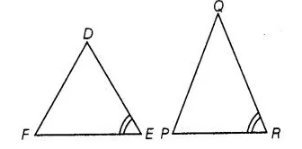
$\therefore$ $\triangle D E F \sim \triangle Q R P$ [by AAA similarity criterion]
$\Rightarrow$ $\angle F=\angle P$ [corresponding angles of similar triangles]
$\therefore$ $\frac{D F}{Q P}=\frac{E D}{R Q}=\frac{F E}{P R}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
