If perpendiculars from any point within an angle on its arms are congruent.

Given that, if perpendicular from any point within, an angle on its arms is congruent, prove that it lies on the bisector of that angle.

Now,

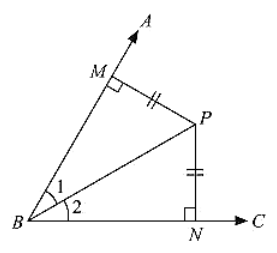
Let us consider an angle ABC and let BP be one of the arm within the angle

Draw perpendicular PN and PM on the arms BC and BA such that they meet BC and BA in N and M respectively.

Now, in ΔBPM and ΔBPN

We have ∠BMP = ∠BNP = 90° [given]

BP = BP [Common side]

And MP = NP [given]

So, by RHS congruence criterion, we have

ΔBPM ≅ ΔBPN

Now, ∠MBP = ∠NBP [Corresponding parts of congruent triangles we equal]

BP is the angular bisector of ∠ABC.

Hence proved