If $\sin A=\frac{\mathbf{3}}{\mathbf{4}}$, calculate $\cos A$ and $\tan A$.

Solution:

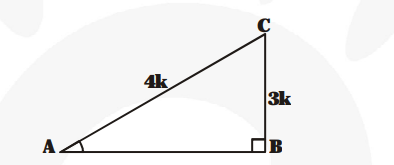
In figure,

$\sin A=\frac{3}{4}$

$\Rightarrow \frac{B C}{A C}=\frac{3}{4}$

$\Rightarrow \mathrm{BC}=3 \mathrm{k}$

and $A C=4 k$

where k is the constant of proportionality.

By Pythagoras Theorem,



$\mathrm{AB}^{2}=\mathrm{AC}^{2}-\mathrm{BC}^{2}=(4 \mathrm{k})^{2}-(3 \mathrm{k})^{2}=7 \mathrm{k}^{2}$

$\Rightarrow \mathrm{AB}=\sqrt{7} \mathrm{k}$

So, $\cos A=\frac{A B}{A C}=\frac{\sqrt{7 k}}{4 k}=\frac{\sqrt{7}}{4}$

and $\tan \mathrm{A}=\frac{\mathbf{B C}}{\mathbf{A B}}=\frac{\mathbf{3 k}}{\sqrt{\mathbf{7 k}}}=\frac{\mathbf{3}}{\sqrt{\mathbf{7}}}$