If the distance of the point $(1,-2,3)$ from the plane $x+2 y-3 z+10=0$ measured parallel to the line, $\frac{x-1}{3}=\frac{2-y}{m}=\frac{z+3}{1}$ is $\sqrt{\frac{7}{2}}$, then the value of $|m|$ is equal to______.
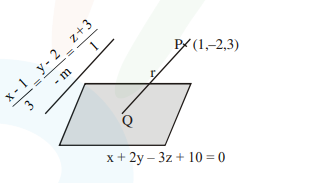
$\mathrm{DC}$ of line $\equiv\left(\frac{3}{\sqrt{\mathrm{m}^{2}+10}}, \frac{-\mathrm{m}}{\sqrt{\mathrm{m}^{2}+10}}, \frac{1}{\sqrt{\mathrm{m}^{2}+10}}\right)$
$Q \equiv\left(1+\frac{3 r}{\sqrt{m^{2}+10}},-2+\frac{-m r}{\sqrt{m^{2}+10}}, 3+\frac{r}{\sqrt{m^{2}+10}}\right)$
Q lies on $x+2 y-3 z+10=0$
$1+\frac{3 \mathrm{r}}{\sqrt{\mathrm{m}^{2}+10}}-4-\frac{2 \mathrm{mr}}{\sqrt{\mathrm{m}^{2}+10}}-9-\frac{3 \mathrm{r}}{\sqrt{\mathrm{m}^{2}+10}}+10=0$
$\Rightarrow \frac{r}{\sqrt{m^{2}+10}}(3-2 m-3)=2$
$\Rightarrow \frac{r}{\sqrt{m^{2}+10}}(-2 m)=2$
$\mathrm{r}^{2} \mathrm{~m}^{2}=\mathrm{m}^{2}+10$
$\frac{7}{2} m^{2}=m^{2}+10 \Rightarrow \frac{5}{2} m^{2}=10 \Rightarrow m^{2}=4$
$|\mathrm{m}|=2$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
