If the median of the distribution given below is 28.5,

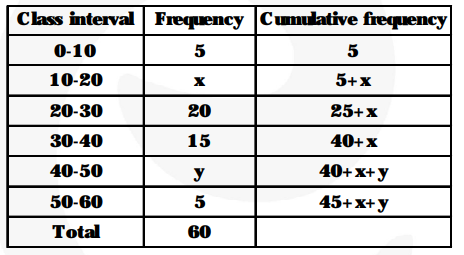
Median = 28.5 lies in the class-interval (20-30). 

Then median class is (20-30).

So, we have $\ell=20, \mathrm{f}=20, \mathrm{cf}=5+\mathrm{x}, \mathrm{h}=10, \mathrm{n}=60$

Median $=\ell+\left\{\frac{\frac{\mathbf{n}}{\mathbf{2}}-\mathbf{c f}}{\mathbf{f}}\right\} \times \mathrm{h}=28.528 .5=20+\left\{\frac{\mathbf{3 0}-\mathbf{( 5}+\mathbf{x})}{\mathbf{2 0}}\right\} \times 10$

$\Rightarrow 8.5=\frac{\mathbf{2 5}-\mathbf{x}}{\mathbf{2}} \Rightarrow 17=25-x \Rightarrow x=8$

Find the given table, we have

i.e., x + y + 45 = 60 or x + y = 15

$\Rightarrow y=15-x=15-8=7$, i.e., $y=7$