If the solve the problem

Given : $f(x)=2 \sin x-x$

$\Rightarrow f^{\prime}(x)=2 \cos x-1$

For a local maximum or a local minimum, we must have

$f^{\prime}(x)=0$

$\Rightarrow 2 \cos x-1=0$

$\Rightarrow \cos x=\frac{1}{2}$

$\Rightarrow x=\frac{\pi}{3}$ or $\frac{-\pi}{3}$

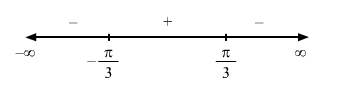
Since $f^{\prime}(x)$ changes from positive to negative when $x$ increases through $\frac{\pi}{3}, x=\frac{\pi}{3}$ is the point of local maxima. The local maximum value of $f(x)$ at $x=\frac{\pi}{3}$ is given by

$2 \sin \left(\frac{\pi}{3}\right)-\frac{\pi}{3}=\sqrt{3}-\frac{\pi}{3}$

Since $f^{\prime}(x)$ changes from negative to positive when $x$ increases through $-\frac{\pi}{3}, x=-\frac{\pi}{3}$ is the point of local minima.

The local minimum value of $f(x)$ at $x=-\frac{\pi}{3}$ is given by

$2 \sin \left(\frac{-\pi}{3}\right)+\frac{\pi}{3}=\frac{\pi}{3}-\sqrt{3}$