If $z_{1}, z_{2}$ are complex numbers such that $\operatorname{Re}\left(\mathrm{z}_{1}\right)=\left|\mathrm{z}_{1}-1\right|, \operatorname{Re}\left(\mathrm{z}_{2}\right)=\left|\mathrm{z}_{2}-1\right|$ and
$\arg \left(z_{1}-z_{2}\right)=\frac{\pi}{6}$, then $\operatorname{Im}\left(z_{1}+z_{2}\right)$ is equal to:
Correct Option: , 4
$\operatorname{Re}(z)=|z-1|$
$\Rightarrow \quad x=\sqrt{(x-1)^{2}+(y-0)^{2}} \quad(x>0)$
$\Rightarrow \quad y^{2}=2 x-1=4 \cdot \frac{1}{2}\left(x-\frac{1}{2}\right)$
$\Rightarrow$ a parabola with focus $(1,0) \&$ directrix as
imaginary axis.
$\therefore \quad$ Vertex $=\left(\frac{1}{2}, 0\right)$
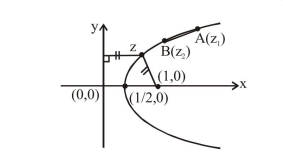
$\mathrm{A}\left(\mathrm{z}_{1}\right) \& \mathrm{~B}\left(\mathrm{z}_{2}\right)$ are two points on it such that
slope of $\mathrm{AB}=\tan \frac{\pi}{6}=\frac{1}{\sqrt{3}}$
$\left(\arg \left(\mathrm{z}_{1}-\mathrm{z}_{2}\right)=\frac{\pi}{6}\right)$
for $\quad y^{2}=4 a x$
Let $\mathrm{A}\left(\mathrm{at}_{1}{ }^{2}, 2 \mathrm{at}_{1}\right) \& \mathrm{~B}\left(\mathrm{at}_{2}{ }^{2}, 2 \mathrm{at}_{2}\right)$
$\mathrm{m}_{\mathrm{AB}}=\frac{2}{\mathrm{t}_{1}+\mathrm{t}_{2}}=\frac{4 \mathrm{a}}{\mathrm{y}_{1}+\mathrm{y}_{2}}=\frac{1}{\sqrt{3}}$
$\left(\right.$ Here $\left.\mathrm{a}=\frac{1}{2}\right)$
$\Rightarrow \quad \mathrm{y}_{1}+\mathrm{y}_{2}=4 \mathrm{a} \sqrt{3}=2 \sqrt{3}$
