If the zeros of the polynomial f(x)

Let $\alpha=a-d, \beta=a$ and $\gamma=a+d$ be the zeros of the polynomial

$f(x)=2 x^{3}-15 x^{2}+37 x-30$

Therefore

$\alpha+\beta+\gamma=\frac{\text { Coefficient of } x^{2}}{\text { Coefficient of } x^{3}}$

$=-\left(\frac{-15}{2}\right)$

$=\frac{15}{2}$

$\alpha \beta \gamma=\frac{-\text { Constant term }}{\text { Coefficient of } x^{2}}$

$=-\left(\frac{-30}{2}\right)$

= 15

Sum of the zeros $=\frac{\text { Coefficient of } x^{2}}{\text { Coefficient of } x^{3}}$

$(a-d)+a+(a+d)=\frac{15}{2}$

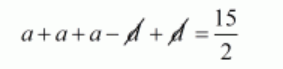
$3 a=\frac{15}{2}$

$a=\frac{5}{2}$

Product of the zeros $=\frac{-\text { Constant term }}{\text { Coefficient of } x^{2}}$

$\alpha \beta \gamma=15$

$(a-d)+a+(a+d)=15$

$a\left(a^{2}-d^{2}\right)=15$

Substituting $a=\frac{5}{2}$ we get

$\frac{5}{2}\left(\left(\frac{5}{2}\right)^{2}-d^{2}\right)=15$

$\frac{5}{2}\left(\frac{25}{4}-d^{2}\right)=15$

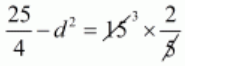
$\frac{25}{4}-d^{2}=3 \times 2$

$\frac{25}{4}-d^{2}=6$

$-d^{2}=6-\frac{25}{4}$

$-d^{2}=\frac{24-25}{4}$

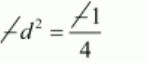
$d \times d=\frac{1}{2} \times \frac{1}{2}$

$d=\frac{1}{2}$

Therefore, substituting $a=\frac{5}{2}$ and $d=\frac{1}{2}$ in $\alpha=a-d, \beta=a$ and $\gamma=a+d$

$\alpha=a-d$

$\alpha=\frac{5}{2}-\frac{1}{2}$

$\alpha=\frac{5-1}{2}$

$\alpha=\frac{4}{2}$

$\alpha=2$

$\beta=a$

$\gamma=a+d$

$\gamma=\frac{5}{2}+\frac{1}{2}$

$\gamma=\frac{5+1}{2}$

$\gamma=\frac{6}{2}$

$y=3$

Hence, the zeros of the polynomial are

$2, \frac{5}{2}, 3$