Question:
If x + y + z = 0, prove that
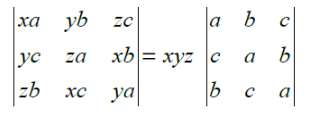
Solution:
$\begin{aligned}&\text { Taking, } \\&\text { L.H.S. }\end{aligned}=\left|\begin{array}{lll}x a & y b & z c \\ y c & z a & x b \\ z b & x c & y a\end{array}\right|$
[Expanding]
$=x a\left(a^{2} y z-x^{2} b c\right)-y b\left(y^{2} a c=b^{2} x z\right)+z c\left(c^{2} x y-z^{2} a b\right)$
$=x y z a^{3}-x^{3} a b c-y^{3} a b c+b^{3} x y z+c^{3} x y z-z^{3} a b c$
$=x y z\left(a^{3}+b^{3}+c^{3}\right)-a b c\left(x^{3}+y^{3}+z^{3}\right)$
$=x y z\left(a^{3}+b^{3}+c^{3}\right)-a b c(3 x y z)$
$\left[\because x+y+z=0 \Rightarrow x^{3}+y^{3}+z^{3}-3 x y z\right]$
$=x y z\left(a^{3}+b^{3}+c^{3}-3 a b c\right)$
$=x y z\left|\begin{array}{lll}a & b & c \\ c & a & b \\ b & c & a\end{array}\right|=\mathbf{R . H . S}$
Hence proved.
