In a circular table cover of radius 32 cm,

Solution:

$\mathrm{O}$ is the centre of the circular table cover and radius $=32 \mathrm{~cm} . \triangle \mathrm{ABC}$ is equilateral. Join $\mathrm{OA}, \mathrm{OB}, \mathrm{OC}$.

Now, $\angle \mathrm{AOB}=\angle \mathrm{BOC}=\angle \mathrm{COA}=120^{\circ}$

In $\Delta \mathrm{OBC}$, we have $\mathrm{OB}=\mathrm{OC}$

Draw $\mathrm{OM} \perp \mathrm{BC}$.

$\Rightarrow \angle \mathrm{BOM}=\angle \mathrm{COM}=60^{\circ}$

$(\because \Delta \mathrm{OMB} \cong \Delta \mathrm{OMC}$ by RHS congruence $)$

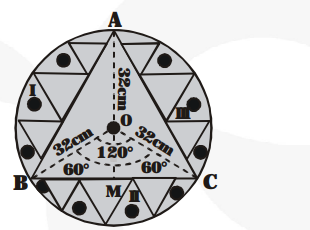
Now, $\frac{\text { BM }}{\text { OB }}=\sin \mathbf{6 0}^{\circ}$

$\Rightarrow \frac{\mathbf{B M}}{\mathbf{3 2}}=\frac{\sqrt{\mathbf{3}}}{\mathbf{2}}$

$\Rightarrow \frac{\mathbf{B M}}{\mathbf{3 2}}=\frac{\sqrt{\mathbf{3}}}{\mathbf{2}}$

$\Rightarrow \mathrm{BM}=\mathbf{1 6} \sqrt{\mathbf{3}} \mathrm{cm}$

Then, BC = 2 × BM

$=32 \sqrt{3} \mathrm{~cm}$

Thus, the side of the equilateral triangle $\mathrm{ABC}=\mathbf{3 2} \sqrt{\mathbf{3}} \mathbf{c m}$

The area of the shaded region (designed)

$=$ The area of circle $-$ area of $\triangle \mathrm{ABC}$

$=\left\{\pi \times(32)^{2}-\frac{\sqrt{\mathbf{3}}}{\mathbf{4}} \times(32 \sqrt{\mathbf{3}})^{2}\right\} \mathrm{cm}^{2}$

$=\left\{\frac{22}{7} \times 32 \times 32-\frac{\sqrt{3}}{4} \times 32 \times 32 \times 3\right\} \mathrm{cm}^{2}=\left\{\frac{22528}{7}-768 \sqrt{3}\right\} \mathrm{cm}^{2}$