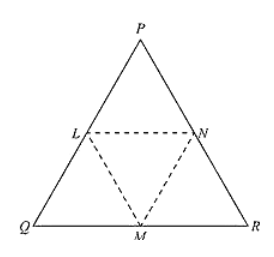
In a PQR, if PQ = QR and L, M and N are the mid-points of the sides PQ, QR and RP respectively.

Given that,

In PQR, PQ = QR and L, M, N are midpoints of the sides PQ, QP and RP respectively and given to prove that LN = MN

Here we can observe that PQR is an isosceles triangle

PQ = QR and ∠ QPR = ∠ QRP  .... (i)

And also, L and M are midpoints of PQ and QR respectively

PL = LQ = QM = MR = PQ/2 = QR/2

And also, PQ = QR

Now, consider Δ LPN and Δ MRN, LP = MR              [From - (2)]

∠LPN = ∠MRN ... [From - (1)]

∠QPR and ∠LPN and ∠QRP and ∠MRN are same.

PN = NR                                                             [N is midpoint of PR]

So, by SAS congruence criterion, we have ΔLPN = ΔMRN

LN = MN [Corresponding parts of congruent triangles are equal]