In a triangle, the sides are given as 11 cm,

True

Since the sides of a triangle are $a=11 \mathrm{~cm}, b=12 \mathrm{~cm}$ and $c=13 \mathrm{~cm}$.

Now, semi-perimeter, $\quad s=\frac{a+b+c}{2}$

$=\frac{11+12+13}{2}=\frac{36}{2}=18 \mathrm{~cm}$

Area of a triangle $=\sqrt{s(s-a)(s-b)(s-c)}$ [by Heron's formula]

$=\sqrt{18(18-11)(18-12)(18-13)}$

$=\sqrt{18 \times 7 \times 6 \times 5}$

$=\sqrt{3 \times 6 \times 7 \times 6 \times 5}$

$=6 \sqrt{3 \times 7 \times 5}$

$=6 \sqrt{105}=6 \times 10.25$

$=61.5 \mathrm{~cm}^{2}$

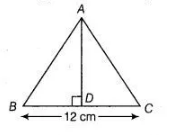
$\therefore \quad$ Area of $\triangle A B C=\frac{1}{2} \times B C \times A D \quad\left[\because\right.$ area of triangle $=\frac{1}{2}$ (base $\times$ height) $]$

$=\frac{1}{2} \times 12 \times 10.25=6 \times 10.25=61.5 \mathrm{~cm}^{2}$