In $\triangle A B C, \angle A=60^{\circ}$. Prove that $B C^{2}=A B^{2}+A C^{2}-A B \cdot A C$.
In $\triangle A B C$ in which $\angle A$ is an acute angle with $60^{\circ}$.
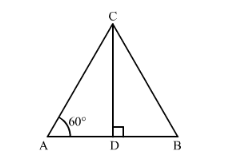
$\sin 60^{\circ}=\frac{C D}{A C}=\frac{\sqrt{3}}{2}$
$\Rightarrow C D=\frac{\sqrt{3}}{2} A C$....(1)
$\cos 60^{\circ}=\frac{A D}{A C}=\frac{1}{2}$
$\Rightarrow A D=\frac{1}{2} A C$.....$(2)$
Now apply Pythagoras theorem in triangle BCD
$B C^{2}=C D^{2}+B D^{2}$
$=C D^{2}+(A B-A D)^{2}$
$=\left(\frac{\sqrt{3}}{2} A C\right)^{2}+A B^{2}+\left(\frac{1}{2} A C\right)^{2}-2 A B \frac{1}{2} A C$
$=A C^{2}+A B^{2}-A B \times A C$
Hence $B C^{2}=A B^{2}+A C^{2}-A B \cdot A C$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
