In an equailateral triangle ABC,

Solution:

AB = BC = CA = a (say)

$B D=\frac{1}{3} B C=\frac{1}{3} a$

$\Rightarrow C D=\frac{2}{3} B C=\frac{2}{3} a$



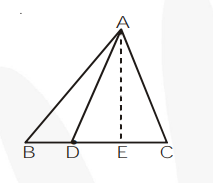
$\mathrm{AE} \perp \mathrm{BC}$

$\Rightarrow B E=E C=\frac{1}{2} a$

$\mathrm{DE}=\frac{1}{2} \mathrm{a}-\frac{1}{3} \mathrm{a}=\frac{1}{6} \mathrm{a}$

$\Delta \mathrm{D}^{2}=\Delta \mathrm{F}^{2}+\mathrm{DF}^{2}=\Delta \mathrm{B}^{2}-\mathrm{BF}^{2}+\mathrm{DF}^{2}$

$=a^{2}-\left(\frac{1}{2} a\right)^{2}+\left(\frac{1}{6} a\right)^{2}$

$=a^{2}-\frac{1}{4} a^{2}+\frac{1}{36} a^{2}$

$=\frac{(36-9+1) a^{2}}{36}=\frac{28}{36} a^{2}=\frac{7}{9} A B^{2}$

$\Rightarrow 9 \mathrm{AD}^{2}=7 \mathrm{AB}^{2}$