In fig., a square OABC is inscribed in a quadrant OPBQ.

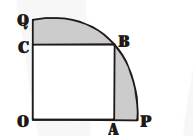
OABC is a square such that its side OA = 20 cm

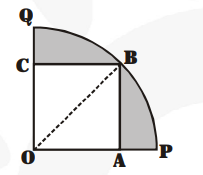
$\therefore \mathrm{OA}=20 \mathrm{~cm}$

$\therefore \mathrm{OB}^{2}=\mathrm{OA}^{2}+\mathrm{AB}^{2}$

$\therefore \mathrm{OB}^{2}=20^{2}+20^{2}$

$=400+400=800$

$\mathrm{OB}=\sqrt{\mathbf{8 0 0}}=\mathbf{2 0} \sqrt{\mathbf{2}} \mathrm{cm}$

Now, area of the quadrant $\mathrm{OPBQ}=\frac{1}{4} \pi \mathrm{r}^{2}$

$=\frac{\mathbf{1}}{\mathbf{8}} \times \frac{\mathbf{3 1 4}}{\mathbf{1 0 0}} \times \mathbf{8 0 0} \mathrm{cm}^{2}=314 \times 2=628 \mathrm{~cm}^{2}$

Area of the square $\mathrm{OABC}=20 \times 20 \mathrm{~cm}^{2}$

$=400 \mathrm{~cm}^{2}$

$\therefore$ Area of the shaded region $=$ Area of the quadrant $\mathrm{OPBQ}-$ Area of the square $\mathrm{OABC}$

$=628 \mathrm{~cm}^{2}-400 \mathrm{~cm}^{2}=228 \mathrm{~cm}^{2}$