In fig., AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle.

Solution:

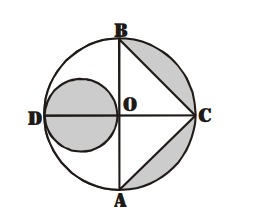
O is the centre of the circle, OA = 7 cm

AB = 2(OA) = 2 × 7 = 14 cm

OC = OA = 7 cm 

$\because \quad \mathrm{AB}$ and $\mathrm{CD}$ are perpendicular to each other

$\Rightarrow \mathrm{OC} \perp \mathrm{AB}$

$\therefore \quad$ Area of $\Delta \mathrm{ABC}$

$=\frac{1}{2} \times \mathrm{AB} \times \mathrm{OC}=\frac{1}{2} \times 14 \mathrm{~cm} \times 7 \mathrm{~cm}=49 \mathrm{~cm}^{2}$

Again OD = OA = 7 cm

$\therefore$ Radius of the small circle

$=\frac{1}{2}(\mathrm{OD})=\frac{1}{2} \times 7=\frac{7}{2} \mathrm{~cm}$

$\therefore \quad$ Area of the small circle $=\pi \mathrm{r}^{2}$

$=\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2} \mathrm{~cm}^{2}=\frac{11 \times 7}{2}=\frac{77}{2} \mathrm{~cm}^{2}$

Radius of the big circle $=\frac{\mathbf{1 4}}{\mathbf{2}} \mathrm{cm}=7 \mathrm{~cm}$

Area of the semi-circle $\mathrm{OACB}=\frac{\mathbf{1}}{\mathbf{2}} \pi \mathrm{r}^{2}$

$=\frac{\mathbf{1}}{\mathbf{2}}\left(\frac{\mathbf{2 2}}{\mathbf{7}} \times \mathbf{7} \times \mathbf{7}\right) \mathbf{m m}^{\mathbf{2}}=11 \times 7 \mathrm{~cm}^{2}=77 \mathrm{~cm}^{2}$

Now, Area of the shaded region

$=[$ Area of the small circle $]+[$ Area of the big semi-circle $\mathrm{OABC}]-[$ Area of $\triangle \mathrm{ABC}]$

$=\frac{77}{2} \mathrm{~cm}^{2}+77 \mathrm{~cm}^{2}-49 \mathrm{~cm}^{2}$

$=\frac{77+154-98}{2} \mathrm{~cm}^{2}$

$=\frac{231-98}{2} \mathrm{~cm}^{2}=\frac{133}{2} \mathrm{~cm}^{2}=66.5 \mathrm{~cm}^{2}$