In fig., $\mathrm{XY}$ and $\mathrm{X}^{\prime} \mathrm{Y}^{\prime}$ are two parallel tangents to a circle with centre $\mathrm{O}$ and another tangent $\mathrm{AB}$ with point of contact $\mathrm{C}$ intersecting $\mathrm{XY}$ at $\mathrm{A}$ and $\mathrm{X}^{\prime} \mathrm{Y}^{\prime}$ at $\mathrm{B}$. Prove that $\angle \mathrm{AOB}=90^{\circ}$
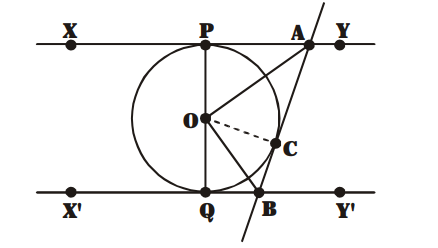
In fig., Join OC and we have $\Delta \mathrm{s} \mathrm{AOP}$ and $\mathrm{AOC}$ for which
AP = AC (Both tangents from A)
OP = OC (Each = radius)
OA = OA (Common side)
$\Rightarrow \quad \Delta A O P \cong \Delta A O C(S S S$ congruence $)$
$\Rightarrow \quad \angle \mathrm{PAO}=\angle \mathrm{CAO}$
$\Rightarrow \quad \angle \mathrm{PAC}=2 \angle \mathrm{OAC} \quad \ldots(1)$
Similarly, $\angle \mathrm{QBC}=2 \angle \mathrm{OBC} \ldots(2)$
Adding (1) and (2),
$\angle \mathrm{PAC}+\angle \mathrm{QBC}=2\{\angle \mathrm{OAC}+\angle \mathrm{OBC}\}$
$\Rightarrow 180^{\circ}=2\{\angle \mathrm{OAC}+\angle \mathrm{OBC}\}$
$\left(\because\right.$ in quadrilateral $\left.\mathrm{PABQ}, \angle \mathrm{P}=\angle \mathrm{Q}=90^{\circ}\right\}$
$\Rightarrow \angle \mathrm{OAC}+\angle \mathrm{OBC}=\frac{\mathbf{1}}{\mathbf{2}} \times 180^{\circ}=90^{\circ} \ldots(3)$
Now, in $\triangle \mathrm{AOB}$ we have
$\angle \mathrm{AOB}+\angle \mathrm{OAC}+\angle \mathrm{OBC}=180^{\circ}$
$\Rightarrow \angle \mathrm{AOB}+90^{\circ}=180^{\circ} \quad(\mathrm{By}(3))$
$\Rightarrow \angle \mathrm{AOB}=90^{\circ}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
